The Theory Group

Physics in High Magnetic Fields

I.D.Vagner

Grenoble High Magnetic Field Laboratory (CNRS-MPI)
166X, F-48042, Grenoble, Cedex 9, France.
Tel: +33-4-76-88-11-27; Fax: +33-4-76-85-56-10.
E-Mail: vagner@labs.polycnrs-gre.fr

Physics and Engineering Research Institute (P.E.R.I.)
Ruppin Instutute for Higher Education
Emek Hefer 40250, Israel.
Tel: (+972)-9898-1340, (+972)-9898-3042;
Fax: (+972)-9898-6848
E-mail: perinst@netvision.net.il


Mesoscopics

  1. Electrons on a nanosphere
  2. Spin-Flip Assisted Resonant Tunnelling
  3. Hyperfine Interaction and Residual Resistivity in Normal and Ferromagnetic Metals

1. Electrons confined on the surface of a sphere in a magnetic field
Simple models have often served as useful paradigms to gain insight into the behavior of realistic systems. A notable example is the energy spectrum of a free electron in a magnetic field (Landau levels) which provides the basis for understanding a wide range of physical phenomena, such as quantum oscillations of thermodynamical and transport properties in metals and semiconductors.
In recent years, magnetotransport in mesoscopic systems attracts sharply growing attention. A long standing problem is an electron in a combined sphericaliy symmetric potential and in a homogeneous magnetic field, which originates in atomic physics (orbital Zeeman effect). In condensed matter physics this has been used for studies of excitons in semiconductors and of small metallic clusters.
We consider the effect of a uniform magnetic field on the quantum mechanical spectrum of non- interacting electrons whose motion is constrained to the surface of a sphere. In the absence of the magnetic field, the problem is fully degenerate and as expected, the field breaks this degeneracy. This leads to interesting level crossing effects in the spectrum which are reflected in the magnetic response of the system.

In figure 3.7 we show the variation of E(l,ls) with magnetic flux for l<9, where the solutions of the continued fractions are obtained numerically. The relative importance of the linear (paramagnetic) versus the quadratic (diamagnetic) contributions leads to a decrease in energy at low fields while an in- crease results at high fields. The critical field where the trend reverses increases with lz.
This level crossing behavior leads to complicated structures in the field dependence of the magnetization figure 3.8. We calculate these properties from the free energy, F = omega-mu, for a fixed number N of electrons on the sphere.

The magnetization for electrons in the ground state oscillates almost periodically with the flux Fi through the equatorial plane of the sphere. The period of these oscillations is close to the flux quantum Fio. The first oscillations indicate variable periodicity, is always larger than Fio, which decreases with the field. We attribute this behavior to the Aharonov-Bohm effect in the spherical geometry where the wave-function is centered away from the equatorial plane. Due to advances in the fabrication technology, it may be possible to measure these interesting physical properties.

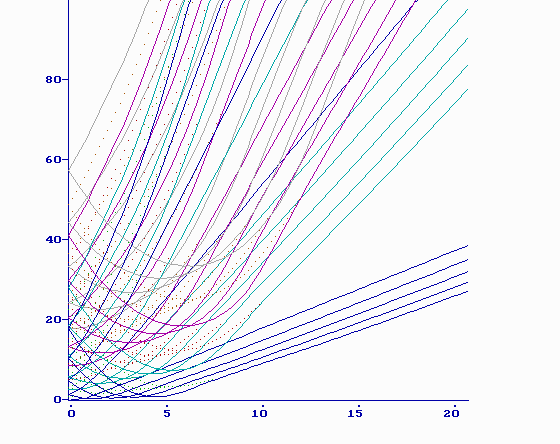
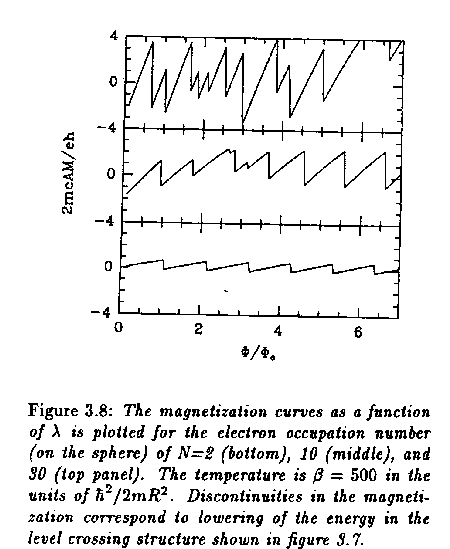
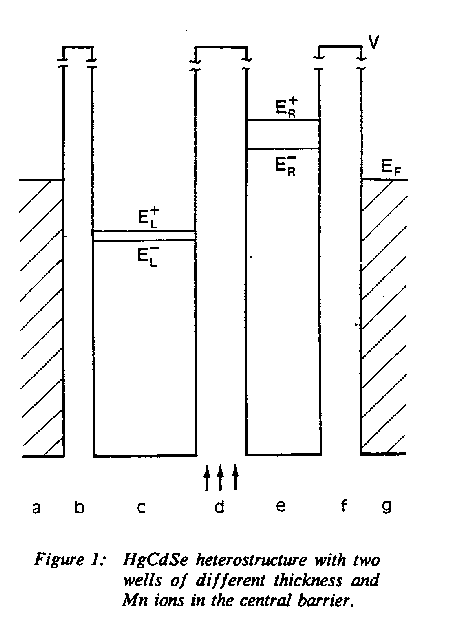
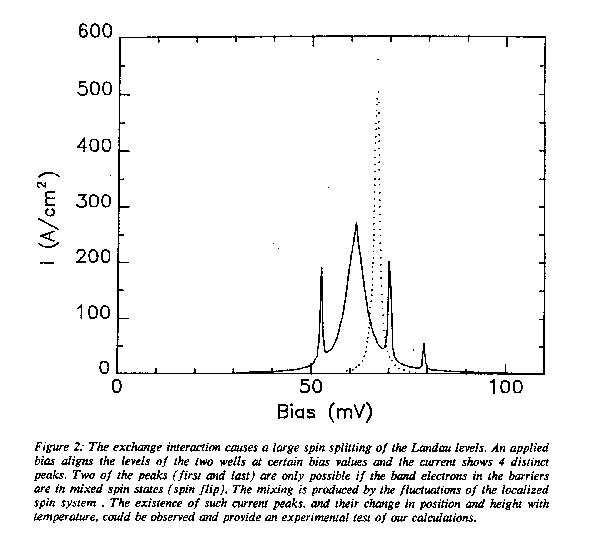
2. Spin-Flip Assisted Resonant Tunnelling

Resonant tunnelling (RT) through quasistationary states in a single quantum well has been the subject of numerous experimental and theoretical work, since it became possible to grow semiconductor heterostructures by molecular beam epitaxy. Recent progress in growing of quantum-wells based on II-VI compounds, such as Cd1-x,Mnx1Te-CdTe has introduced a new set of physical problems, following from a strong interaction between the conduction electrons and the localised spins of the Mn2+ ions. Significant penetration of the carrier wave function in the CdTe quantum well into the barriers with Mn2+ results in a strong influence of the exchange interaction on the electronic properties.

Application of strong magnetic fields results in quantization, with a large degeneracy, in the x and y directions. The resonant tunnelling through one well in a magnetic field has been intensively investigated. Less attention has been paid, however, to double quantum wells, where the energetical alignment of two discrete quasistationary states with a large degeneracy may yield a sharp current peak. The sharp current peaks provide the possibility to investigate such fundamental problems as Landau level broadening in quantum wells and, as we have shown, the exchange interaction between band electrons and magnetic impurities in the barriers.

Since in a semiconductor the electrons have much longer wavelength, than in metals, they interact simultaneously with a large number of magnetic impurities. In this case the classical approximation to the Heisenberg Hamiltonian can be applied and the formalism can be written in a simple way. This allows the application to more compiicated structures than the one barrier case. We have developed a theory of the resonant tunnelling via Zeeman splitted Landau levels in diluted magnetic semiconductor triple barrier heterostructures (see Fig.l). The exchange interaction is included into tunnelling using a transfer matrix technique for spinors.

As a result of the exchange interaction we predict additional resonances in the tunnel current through a triple barrier heterostructure including magnetic impurities. This result may stimulate further investigation on the connection between electronic and magnetic properties of diluted magnetic semiconductor heterostructures. Most interestingly it provides the opportunity to probe the magnetic behaviour of a several Awide layer by a tunnel current and it is of importance in the research on 2D-spin systems.

We show in Fig.2 (dashed line) the tunnel current for the case without Mn ions. The reason for the sharp peak is the alignment of two (highly degenerated) quasistationary states quantized in the x and y direction by a magnetic field and in the z direction by size quantization in each well. We found similar sharp peaks for a GaAs-AlAs heterostructure. The width of the peak can be decreased with thicker barriers and is only limited by the Landau level broadening.

3. MESO-NUCLEO-SPINICS: Hyperfine interaction driven mesoscopic effects

 A family of new physical effects in nanostructures with strong spin-orbital coupling appears when the electron spin degeneracy is lifted by a hyperfine field of polarized nuclei. Indeed, the combined action of a strong nuclear polarization and the spin-orbit interaction, breaks the time reversal symmetry in a mesoscopic system. A good illustration for such meso-nucleo-spinic effects is the modified Aharonov-Bohm effect in mesoscopic rings.
Persistent currents (the diamagnetic moment in mesoscopic nonsuperconducting rings) reflect the broken clock wise-anticlock wise symmetry, caused, traditionally, by the external vector potential, Experimentally this is achieved by application of a time dependent external magnetic field along the ring axis. The magnetic field variation results in the oscillatory, with the Aharonov-Bohm period behavior of the persistent current.
We have shown, however, that in GaAs/AlGaAs quantum ring with a nonequilibrium nuclear spin population, the persistent current will exist even in the absence of external magnetic field . It will oscillate, with time, during the time interval of the order of nuclear spin relaxation time . The physics behind these oscillations can be understood along the following lines. The hyperfine field, caused by the nonequilibrium nuclear spin population breaks the spin symmetry of conduction electrons which, combined with a strong spin - orbital coupling, will result in the breaking of the rotational symmetry of diamagnetic currents in a ring. The oscillations of persistent current arise due to the exponential time dependence of the phase (see Fig.3) with the time constant which was measured to be, in GaAs/AlGaAs of the order of hours at low temperatures.
In order to create a topologically nontrivial effective vector potential in a heterojunction, either the nuclei polarization or the spin-orbit coupling should be inhomogeneous along the perimeter of the ring. Out of a variety of different experimental realizations let us outline the following ones.
Since the nuclear relaxation rate T in GaAs/AlGaAs heterojunction is highly sensitive to the impurities distribution , one can achieve different nuclear polarizations in different parts of a mesoscopic ring, provided the characteristic length of the impurity potential is of order of several hundreds of , i.e. comparable to the ring width. In this configuration we expect that the mesoscopic sensitivity to a single impurity position may produce a nonvanishing phase

Figure 3
Oscillations of persistent current due to the combined action of the hyperfine field and spin-orbit interaction. The aperiodic form reflects the exponential time dependence of the phase .

Another way of obtaining a nonzero circulation of the effective vector potential is creation of the slowly varying on the scale coordinate dependent spin-orbit coupling connected with external potentials like boundaries, heavy atoms impurities along the perimeter of the ring and other imperfections which may locally modify the spin-orbit interaction in these systems.
We outline, that this is the first of a series of ''meso-nucleo-spinic'' effects, which should take the place in different systems with broken symmetry due to the combined action of the hyperfine field and spin-orbital interaction.


  1. R. Wessel and I.D. Vagner ''Spin-flip Assisted Resonant Tunneling''. Superlattices and Microstructures, 8, 443 (1990).
  2. Ju H. Kim, I.D. Vagner and B. Sundaram ''Electrons Confined on the Surface of a Sphere in a Magnetic Field'' Phys. Rev. B, 46, 9501 (1992).
  3. I.D. Vagner, A.S. Rozhavsky, P. Wyder and A.Yu. Zyuzin ''Is the magnetic field necessary for the Aharonov-Bohm effect in mesoscopics?'' Phys. Rev. Lett. 80, 2417 (1998) .
  4. V. Privman, I.D. Vagner and G. Kventsel''Quantum computing in quantum Hall systems'' Phys.Lett. A, 239, 141 (1998)



Main Page Visitors Projects Links




Last updated 19-Jan-01.
WebMaster AK